DIVERGENCES OF INTEGRALS FOR GREEN FUNCTIONS OF KLEIN-GORDON AND DIRAC EQUATIONS AND NECESSARY EXISTENCE OF PARTICLE GENERATIONS

Yu.V. Kulish, E.V. Rybachuk

Full Text :   (600 kB, Eng.) 

Abstract
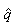It is shown that the values of the infinite integrals for the Green functions of the Klein - Gordon and Dirac equations depend on the method used for its calculations, i.e., these integrals diverge. The Green functions proposed to eliminate these divergences include in the denominators the polynomials of the N degree instead of one factor m²-q² or m- for the Klein - Gordon equation or the Dirac equation, respectively. The corresponding generalizations of the Klein - Gordon and Dirac equations have 2N and N degree, respectively. The solutions of these generalizations for the Klein - Gordon and Dirac equations may be presented by the sum of N terms, each term corresponds to the contribution of one particle (one generation). All these particles have different masses but the same spin, parity, charge, isospin. Since the space - time is 4 - dimensional one, the convergence of the integrals for proposed Green functions is possible only if the generation number N is not less than three for integer spin particles and not less than five for half - integer spin particles. It is shown that the proposed Green functions have no any singularities in the space - time. In particular, the interaction potentials must have the oscillator form at short distance. It is predicted that two (or greater) massive particles with quantum numbers of the photon and gluons must exist. Besides, five (or greater) fermions with quantum numbers of the electron, the neutrino, the quark, and quark must exist (e.g., e₁=e, e₂=μ, e₃=τ, e₄, e₅,... and u₁=u, u₂=c, u₃=t, u₄, u₅,...). The massless neutrino must be one. If higher neutrinos are enough heavy then the decay ν_4,5 → eμν_1,2 may be possible.

KEY WORDS: convergence, multiple integrals, Green functions, partial differential equations, oscillatory potentials, particle generations, massive photon, massive gluons, massive neutrino.

THE NUCLEAR GAMMA-RESONANCE IN RESEARCH OF GRAVITATION AND RELATIVISTIC UNIFORMLY ACCELERATED MOTION. DETECTING OF MOSSBAUER SPECTRA OF ROTATING OBJECTS

V.G. Kirichenko, О.V. Кovalenko, V.N. Leonov, Т.А. Коvalenko, Yu.I. Gofman

Full Text :   (560 kB, Rus.) 

Abstract
Main principles of the effects observation described by the theory of a relativity and gravitation in terrestrial conditions are considered. The reasons of need for carrying out of repeated experiments at higher level of accuracy (above 1 %), on validation and verification purpose of measurable effects are stated. The method of detecting nuclear γ-resonant spectra of rotating objects is offered. A comparison of experimental results obtained by different authors was carried out; the possible reasons for the observed differences in data were discussed and illustrated.

KEY WORDS: M?ssbauer spectroscopy, gravitational redshift, relativistic uniformly accelerated motion.

THEORETICAL MODEL DESCRIBING GROWTH OF SINGLE-WALLED CARBON NANOTUBES IN PLASMA

G.P. Burmaka, I.B. Denysenko, N.A. Azarenkov, R.O. Trofymenko

Full Text :   (450 kB, Rus.) 

Abstract
The surface diffusion model is developed, which describes the growth of forest of single-walled carbon nanotubes in plasma-enhanced chemical vapor deposition. Using the model, the growth rate of nanotubes, diffusion length and residence time of carbon atoms on nanotube surfaces are determined, as functions of external parameters. The model accounts for nonuniformity in deposition of neutral particles on surface of nanotubes from discharge chamber, interactions of hydrocarbon molecules and carbon atoms with etching gas, thermal and ion-induced dissociation of hydrocarbon molecules adsorbed on surface of nanotubes, decomposition of hydrocarbon ions on nanotube surface, as well as diffusion of carbon atoms on surface of nanotubes.

KEY WORDS: carbon nanotubes, growth rate, plasma-enhanced chemical deposition.

THE FUSION PRODUCT LOSSES DUE TO RESONANT MAGNETIC PERTURBATIONS IN TOROIDAL PLASMAS

Yu.K. Moskvitina, A.O. Moskvitin, O.A. Shyshkin

Full Text :   (210 kB, Eng.) 

Abstract
The suppression of edge-localized modes (ELMs) by means of the externally applied resonant magnetic field perturbations (RMPs) and its effect on plasma transport is investigated actively on modern tokamaks. In present paper the modification of loss rates of fusion born alpha particles caused by application of RMPs in tokamak plasma is examined. This study has been performed by means of test-particle simulations. To simplify calculations we use magnetic field model with circular magnetic surfaces. The transport properties of alpha particles are investigated during 3 seconds time interval by tracing the test-particle ensemble. Each particle trajectory is calculated by means of integration of full orbit equations. Three regimes of particle losses are identified during the evolution of the particle ensemble. The formation of magnetic islands together with the stochastic magnetic layers at the plasma edge is the natural consequence of RMPs excitation. It is demonstrated that due to the formation of these resonant magnetic field structures the irregularities of energetic alpha particle orbits occur, and hence the substantial increasing of the losses from the plasma periphery is observed. RMPs slightly affect the first orbit losses of fusion alphas.

KEY WORDS: RMPs, fusion product losses, full orbit, alpha particle.

PHOTO- AND ELECTROFISSION OF TUNGSTEN NUCLEY IN THE ORIENTED SINGLE CRYSTAL

V.I. Kasilov

Full Text :   (215 kB, Rus.) 

Abstract
The paper is concerned with the possibility of using the reactions of photo- and electro-fission of tungsten nuclei as tools to investigate the dynamics of ultrarelativistic electron radiation processes in the oriented single crystal. For this purpose, analysis has been made of the experimental data for orientation dependences of the yields of photo- and electro- fission fragments from single-crystal tungsten in the interaction of 1200 MeV electrons. Consideration has been given to the model of a single crystal represented as a sandwich consisting of two near-surface layers being at the electron beam input into the single crystal and at the crystal output. The fission fragments escape from the layers under the action of photons and electrons. Between the layers, there is the bremsstrahlung radiator representing a part of the single crystal, from which the fission fragments cannot escape because of their short path lengths in the given material. On the basis of the model, the ratio of photofission fragment yields to the electrofission cross-section has been calculated. The resulting ratio has been plotted as a function of the crystal axis orientation angle relative to the direction of the ultrarelativistic electron beam incident on the single crystal. Based on the function, the conclusion has been made about redistribution of channeled and above-barrier particle fluxes in the crystal in the (0-2) mrad range of angles of crystal axis rotation relative to the incident beam direction. It has been also concluded that the bremsstrahlung spectrum in the given angular range also varies, i.e., with crystal rotation in the (0-1) mrad angular range it becomes harder; while at larger angles of disorientation when the crystal becomes equivalent to an amorphous target, the bremsstrahlung spectrum becomes closer to the Schiff spectrum.

KEY WORDS: electron beam, channeling, orientation dependence, dynamics, over-barrier emission

HALL A MOLLER POLARIMETER (JEFFERSON LAB) WITH ELECTRON TARGET POLARIZED IN FOIL PLANE

O.V. Glamazdin, R.I. Pomatsalyuk, E.A. Chudakov

Full Text :   (1100 kB, Rus.) 

Abstract
A polarized electron target with polarization in plane of a foil was used in the Hall A Moller polarimeter (Jefferson Laboratory) in period from 2005 through 2009 year. The target holder consisted of set of six foils with different thicknesses and made of different materials. The target design allowed scanning of every target along and transverse the electron beam of accelerator CEBAF. As the result of using the unique target design accuracy of the electron beam polarization measurements with the M?ller polarimeter became twice better.

KEY WORDS: Moller polarimeter, polarized electron target, polarized electron beam.

MODEL VERIFICATION OF THE SEMICONDUCTOR GAMMA-RAY DETECTORS

A.A. Zakharchenko

Full Text :   (320 kB, Rus.) 

Abstract
Kolmogorov-Smirnov test is used for evaluation of the goodness of fit of amplitude spectra calculated with the previously developed model of wide-gap semiconductor gamma-radiation detector. It was shown that the major part of the amplitude spectra from the 241Am, 152Eu and 137Cs radiation sources are correctly reconstructed. Simplification of the measuring system geometry is a main reason of significant differences between theoretical and experimental response functions. The fluctuations of defect distribution in the detector volume should be included in the detector model to explain the systematic differences at the Compton section of spectrum.

KEY WORDS: detector, response function, Kolmogorov-Smirnov goodness-of-?t test, significance level.

PROPERTIES OF SUPERHARD NANOSTRUCTURED COATINGS TI-HF-SI-N

A.D. Pogrebnjak, A.P. Shpak, V.M. Beresnev, F.F. Komarov, P. Konarski, V.V. Uglov, M.V. Kaverin, N.A. Makhmudov, I. Yakyschenko, B. Postolny, V.V. Grudnitskii, D.A. Kolesnikov

Full Text :   (950 kB, Eng.) 

Abstract
New superhard coatings based on Ti-Hf-Si-N featuring high physical and mechanical properties were fabricated. We employed a vacuum-arc source with HF stimulation and a cathode sintered from Ti-Hf-Si. Nitrides were fabricated using atomic nitrogen (N) or a mixture of Ar/N, which were leaked-in a chamber at various pressures and applied to a substrate potentials. RBS, SIMS, GT-MS, SEM with EDXS, XRD, and nanoindentation were employed as analyzing methods of chemical and phase composition of thin films. We also tested tribological and corrosion properties. The resulting coating was a two-phase, nanostructured nc-(Ti, Hf)N and α-Sі₃N₄. Sizes of substitution solid solution nanograins changed from 3.8 to 6.5 nm, and an interface thickness surrounding α-Si₃N₄varied from 1.2 to 1.8 nm. Coatings hardness, which was measured by nanoindentation was from 42.7 GPa to 48.6 GPa, and an elastic modulus was E = (450 to 515) GPa. The films stoichiometry was defined for various deposition conditions. It was found that in samples with superhard coatings of 42.7 to 48.6GPa hardness and lower roughness in comparison with other series of samples, friction coefficient was equal to 0.2, and its value did not change over all depth (thickness) of coatings. A film adhesion to a substrate was essentially high and reached 25MPa.

KEY WORDS: superhard, nanostructured coatings, Ti-Hf-Si-N, stoichiometry, phase composition.

COEXISTENCE OF VARIOUS TYPES OF CONDUCTIVITY IN Y_1-xPR_xBA₂CU₃O_7-δ SINGLE CRYSTALS WITH DIFFERENT CONCENTRATIONS OF PRASEODYMIUM

A.A. Zavgorodniy, R.V. Vovk, M.A. Obolenskii, Z.F. Nazyrov, E. Churyukova

Full Text :   (555 kB, Ukr.) 

Abstract
It is shown, that the increasing of praseodymium concentration in sumples of Y_1-xPr_xBa₂Cu₃O_7-δ leads to enhancement of the localization and realization in the system of the metal - insulator transition, which always precedes the superconducting transition. Increasing the praseodymium concentration leads to a significant shift of the metal - insulator transition to low temperatures.

KEY WORDS: high-temperature superconductivity, metal - insulator transition, doping, YВaCuO single crystals, localization effects.

THE MICROSTRUCTURE AND DISTRIBUTION OF SECOND PHASE PARTICLES IN AUSTENITIC STEELS

V.G. Kirichenko, O.V. Kovalenko, V.N. Leonov

Full Text :   (1300 kB, Rus.) 

Abstract
In the work the research of the influence of the alloying and thermomechanical treatment on distribution and dimensions of the inclusions of the second phases in the steel 18Cr10NiTi are presented. The methodology of the definition of grains' dimensions according to the data presented by optical metallography with use of the reference sample of the alpha-iron and the real steel metallographic section 18Cr10NiTi was developed as a result of carrying out the work. In the steel 18Cr10NiTi structure and in the additionally alloyed steels the carbide and titanium nitride fine-dispersed inclusions are present. The analysis of the built 3D-diagram has shown that the annealing of all the alloy materials on basis of 18Cr10NiTi at 1200 °С for 30 minutes leads to increase of the inclusions' mean dimension to ~ 0.5÷1 μm, while additional annealing at 800 °С for 5 hours leads to the appreciable removal of the distribution form on the dimensions sideways smaller dimensions. The additional annealing of the alloy 18Cr10NiTi+0.04%TiN at 800 °С after the annealing at 1200 °С for 30 minutes leads to recovery of the initial distribution of the inclusions on the dimensions.

KEY WORDS: microstructure, polycrystal, grain, thermomechanical treatment, distribution.

INFLUENCE OF ION IRRADIATION ON THE STRUCTURE SURFACE OF ZIRCONIUM ALLOYS

V.G. Kirichenko, O.V. Kovalenko, V.N. Leonov, S.V. Starostenko

Full Text :   (1170 kB, Rus.) 

Abstract
The results of studying the influence of ion irradiation on the surface structure of the zirconium alloys layers are presented. Irradiation leads to the formation of amorphous phases in a layer of depth up to 0.3 microns. It was found that thermal annealing of the irradiated foils of alloys leads to a two-stage phase transformation, culminating in the formation of crystalline phases. The obtained data are compared with results of neutron irradiation of alloys zircalloy-2 and others, presented in the article in the form of 3D-diagrams. Data verification for model and real alloys possible to identify the characteristic features of the behavior of alloys in the simulation and neutron irradiation.

KEY WORDS: radiation stability, Zirconium, alloys, surface, ion irradiation, amorphisation, crystallization.

RESEARCH OF CHARACTER OF DESTRUCTION AND MECHANICAL PROPERTIES OF HAFNIUM IN AREA OF TEMPERATURES OF 77-673K

I.M. Neklyudov, М.P. Starolat, А.А. Vasilyev, A.A. Parchomenko, S.P. Stetsenko, R.V. Azhazha, V.V. Makarenko, K.V. Kovtun

Full Text :   (4200 kB, Rus.) 

Abstract
The changes of character of destruction and descriptions of durability and plasticity of hafnium of GFE-1 are investigational depending on the temperature of tests at tension in area of temperatures of 77-673К. Deformation in the explored interval of temperatures is carried out by sliding, and the double is practically absent. Destruction at 670К carries viscid character, and with the drop in the temperature of tests changes on транскристаллитное with сколом. Comparison of mechanisms of destruction of deformation of hafnium of GF-1 and iodide hafnium is at low temperatures.

KEY WORDS: hafnium, deformation, mechanism destruction, mechanical properties.

ANOMALOUS TEMPERATURE DEPENDENCE OF SURFACE DIFFUSION IN RESTRICTED SYSTEMS

I.G. Marchenko, I.I. Marchenko

Full Text :   (780 kB, Rus.) 

Abstract
The paper presents the study on the singularities of particle transport in/through the crystalline surface under the external force exposure. It has been established that singularities arising in the atomic flux dispersion can cause the diffusion parameter change in the different- size systems. The temperature dependences of effective diffusion coefficients are calculated. It is shown that the hyperdiffusion mode occurrence leads to the anomalous temperature dependence of particle flux dispersion in the small systems. The effective diffusion coefficient can be increased with temperature decreasing. Such a unusual behavior vanishes as the size of the system increases

KEY WORDS: diffusion, computer simulation, surface, nanosystems, hyperdiffusion.

ON THE NEW APPROACH TO THE DESCRIPTION OF PHASE TRANSFORMATIONS AND FRAGILITY OF STRONGLY NONEQUILIBRIUM SYSTEMS

I.N. Laptev, А.А. Parkhomenko, I.M. Neklyudov

Full Text :   (270 kB, Rus.) 

Abstract
In work the approach offered earlier to the description and research of conditions martensitic transformation and fragility bcc- metals and alloys is advanced. The new cheme of materials destruction in conditions of action spherical tensor of deformation, characteristic for high dozes reactor irradiations is offered. Basic difference of the given treatment is that a primary element destruction is void (micro-cracs) formation as a result of phase martensitic transformations, instead of plastic deformation as it is accepted in all theories known to us. On this basis the new concept of imitation and forecasting radiation embrittlement is offered. It agrees to it, quality of the main requirement is achievement not similarity of defective structure of materials irradiated in reactors and in imitating conditions (as it was considered till now), but necessity of creation as a result of an imitation irradiation, tensile, or cycling deformation, similarity of a deformation-stress condition.

KEY WORDS: phase transformations, martensitic, fragility, imitation of reactor irradiation.